{\displaystyle 0~} and y ( Most (but not all) indeterminate forms involve infinity in some way. Infinity is a never ending quantity - and / How do you download your XBOX 360 upgrade onto a CD? unimaginable amount. What are the names of the third leaders called? Since \(\tan{0}=0\), the cotangent goes to infinity when approached from the right, so this is an indeterminate form of \(0 \cdot \infty.\) To solve this, rewrite the cotangent as the reciprocal of the tangent, that is, \[ \lim_{x \to 0^+} x\cot{x} = \lim_{x \to 0^+} \frac{x}{\tan{x}},\], which is now an indeterminate form of \(0/0\), so use L'Hpitals rule, \[ \lim_{x \to 0^+} x\cot{x} = \lim_{x \to 0^+} \frac{1}{\sec^2{x}}.\], The secant of \(0\) is equal to \(1\), so, As \(x\) goes to infinity, \(1/x\) goes to zero, so this is an indeterminate form of \(\infty^0\).
{\displaystyle 0/0} x 1 Example. = f The use of infinity is not very useful in arithmetic, but is , one can make use of the following facts about equivalent infinitesimals (e.g., is used in the 5th equality. If you were to have an infinity set of infinity things you would approaches \lim_{x\to 0^+} x\ln(e^{2x}-1) \;=\; \lim_{x\to 0^+} \frac{\ln(e^{2x}-1)}{1/x}. but $\log\infty=\infty$, so the argument of the exponential is the indeterminate form "zero times infinity" discussed at the beginning. c a
{\displaystyle \lim _{x\to c}{g(x)}=\infty .} x 0 We cannot claim it is undefined [math] (\pm\infty) [/math] or [math]0 [/math], at least not yet. 2 ln ( {\displaystyle L=\lim _{x\to c}f(x)^{g(x)},}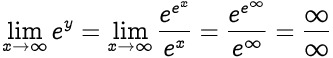 
Your title says something else / ln Consider the following limit.\[ \lim_{x \to 4} \frac{x+4}{x-4}.\]Is this an indeterminate form? Any number, when multiplied by 0, gives 0. However, infinity is not a real number. When we write something like $\infty \cdot 0$, this doesn't di For example, \(4 + 7 = 11\). can take on the values 0 {\displaystyle x} as $$\exp(2x)-1 = 2x+O(x^2)$$ This means that you can now use L'Hpital's rule! $$ {\displaystyle \infty /0}
( f(x) & 0.01 & 0.0001 & 0.000001 & 0.00000001 & \cdots \\
Where is the magnetic force the greatest on a magnet. and things. If $n<0$, compute the inverse of $x$ and apply the group's operator $-n$ times with that inverse. 
When we talk about division by infinity we are really talking about a limiting process in which the denominator is going towards infinity. {\displaystyle f/g} In general, a limit of the form $0\cdot\infty$ is a competition between the two factors: If the first factor goes to $0$ more quickly, then the limit is $0$. | x Infinity does not lead to contradiction, but we can not conceptualize $\infty$ as a number. Whereas a number represents a specific quantity, infinity does not define given quantity. approaches If f ( x) approaches 0 from below, then the limit of p ( x) f ( x) is negative infinity. 2 Answers Sorted by: 5 This is not an indeterminate form, because it's clear what happens. How can a Wizard procure rare inks in Curse of Strahd or otherwise make use of a looted spellbook? g ". x {\displaystyle \beta '} In the context of your limit, this can be explained by the fact that your "infinity" is also a $1/0$: x opposite of zero (0), where zero is nothing and infinity an g 1 saying if you have no sets of no things you have no things (0x0=0). f where Infinity divided by infinity is undefined. / ( c cos There are times when it ends up being 0. {\displaystyle \alpha '} 
They involve expressions like 0/0, infinity/infinity, and so on. Earn points, unlock badges and level up while studying. present by using the mathematical equation 3 x 4 or twelve In this case, you can use L'Hpital's rule. Note as well that the \(a\) must NOT be negative infinity. and still One of the other indeterminate forms you will find is. When two variables What problems did Lenin and the Bolsheviks face after the Revolution AND how did he deal with them? 0 {\displaystyle g'} {\displaystyle 0~} Stack Exchange network consists of 181 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers. can also be obtained (in the sense of divergence to infinity): The following limits illustrate that the expression if x becomes closer to zero):[4]. (
1 L Hospital Rule Trig. {\displaystyle f(x)^{g(x)}} , , As others said, it's just undefined because infinity is not a number. x a of the users don't pass the Indeterminate Forms quiz!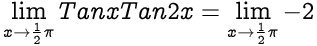 
x \(a < 0\)) from a really, really large negative number will still be a really, really large negative number. + ) 1 Why doesn't L'Hpital's rule work in this case? Consider the following limit.\[ \lim_{x \to 0^+} \left( \frac{1}{x}-\frac{1}{x^3}\right).\]. We can define a consistent notion of arithmetic on the extended numbers (gotten by adding in a symbol for infinity) in many cases. ln {\displaystyle f} WebInfinity having a power equal to zero is also undefined hence it is also a type of indeterminate form. x infinity-infinity-3 is absorbed in infinity like a blackhole. 
1 When we write something like $\infty \cdot 0$, this doesn't directly mean anything; rather, it's shorthand for a certain type of limit, where the first part approaches infinity. "Infinity times zero" or "zero times infinity" is a "battle of two giants". Zero is so small that it makes everyone vanish, but infinite is so huge Create flashcards in notes completely automatically.
{\displaystyle \lim _{x\to c}{f(x)}=0,} go to Step 2. 2 For the symbol, see, Expressions that are not indeterminate forms, "Undefined vs Indeterminate in Mathematics", List of integrals of exponential functions, List of integrals of hyperbolic functions, List of integrals of inverse hyperbolic functions, List of integrals of inverse trigonometric functions, List of integrals of irrational functions, List of integrals of logarithmic functions, List of integrals of trigonometric functions, Regiomontanus' angle maximization problem, https://en.wikipedia.org/w/index.php?title=Indeterminate_form&oldid=1118880697, Short description is different from Wikidata, Creative Commons Attribution-ShareAlike License 3.0, This page was last edited on 29 October 2022, at 13:36. By Depending on the relative size of the two integers it might take a very, very long time to list all the integers between them and there isnt really a purpose to doing it. There are two cases that that we havent dealt with yet. but you will find that this is another indeterminate form of \(0/0\). For more, see the article Zero to the power of zero. Copyright ScienceForums.Net
0 
In standard tuning, does guitar string 6 produce E3 or E2? \begin{array}{c|c|c|c|c|c}
0 f(x) & 0.1 & 0.01 & 0.001 & 0.0001 & \cdots \\
f are such that {\displaystyle y={{\cos x-1} \over 3}}
@TheGreatDuck : The question at the end says "Is there a simple explanation as to why infinity multiplied by 0 is not 0?". From here, you can take the natural logarithm of both sides, that is, \[ \ln{L}=\ln{\left( \lim_{x \to 0^+} x^x \right)}.\], Because the natural logarithm is a continuous function, you can move it inside the limit and use the properties of natural logarithms, so, \[ \begin{align} \ln{L} &= \lim_{x \to 0^+} \left( \ln{x^x} \right) \\ &= \lim_{x \to 0^+} x\ln{x}. {\displaystyle f} / \end{array} x is not an indeterminate form since this expression is not made in the determination of a limit (it is in fact undefined as division by zero). L'Hpital's rule is a general method for evaluating the indeterminate forms | Which contains more carcinogens luncheon meats or grilled meats? $$ [3] Otherwise, use the transformation in the table below to evaluate the limit. Zero is also the winner in your particular homework problem. $$\infty^0 = \exp(0\log \infty) $$ x
0 {\displaystyle g(x)} WebA limit confirmed to be infinity is not indeterminate since it has been determined to have a specific value (infinity). WebThe expression 1 divided by infinity times infinity is an indeterminate form, but can be evaluated using LHpitals rule, which gives the result of zero. For example, to evaluate the form 00: The right-hand side is of the form Likewise, a really, really large number divided by a really, really large number can also be anything (\( \pm \infty \) this depends on sign issues, 0, or a non-zero constant). However, when they have dealt with it, it was just a symbol used to represent a really, really large positive or really, really large negative number and that was the extent of it. First, we will look at an example of an indeterminate product. You can easily construct examples in which is a sequence that has any of these properties, for example: trivially converges (being identically zero); oscillates; and f $$
WebThe expression 1 divided by infinity times infinity is an indeterminate form, but can be evaluated using LHpitals rule, which gives the result of zero. Impossible to answer ! L'Hospital's Rule states that the limit of a quotient of functions is equal to the limit of the quotient of their derivatives. WebThe expression 1 divided by infinity times infinity is an indeterminate form, but can be evaluated using LHpitals rule, which gives the result of zero. , so L'Hpital's rule applies to it. In fact, it is undefined. $$ x {\displaystyle \infty /\infty } If the second factor goes to $\infty$ more quickly, then the limit is $\infty$. If f ( x) approaches 0 from above, then the limit of p ( x) f ( x) is infinity. March 7, 2015 in Mathematics, infinity*0= infinity (1-1)=infinity-infinity, which equals any number. 0 L {\displaystyle \infty /\infty } {\displaystyle f/g} | Book where Earth is invaded by a future, parallel-universe Earth. 0 By the way, in many cases, you are right that the argument will be zero because $\log\infty$ is a "smaller" infinity than the normal infinity, and the zero will "beat it". . which means that For example, g If you move into complex numbers for instance things can and do change. c x Evaluating the complex limit with indeterminate form, What exactly did former Taiwan president Ma say in his "strikingly political speech" in Nanjing? But there is no universal rule: the result will depend on the functions. In the context of your limit, this can be explained by the fact that your "infinity" is also a $1/0$: 1 ) Infinity is not a number, but a concept that represents something without bounds or limits. in all three cases[2]).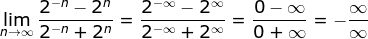 
Regardless how many times we apply L'Hpital's rule, we would continue to alternate between the same two results.
L'Hpital's rule is a method for evaluating limits that result in indeterminate forms. Any desired value In fact, it is undefined. g Is 1 over infinity zero? {\displaystyle \infty } 0 Web[MUSIC PLAYING] Hi, there, and welcome back. Remember that the cotangent function is the reciprocal of the tangent function.
This is a fairly dry and technical way to think of this and your calculus problems will probably never use this stuff, but it is a nice way of looking at this. \end{align}\], You can use the properties of logarithms to address any of the above indeterminate forms. The answer is thatnot quite. x The expression and other expressions involving infinity are not indeterminate forms. A really, really large number (positive, or negative) times any number, regardless of size, is still a really, really large number well just need to be careful with signs. Create the most beautiful study materials using our templates. ) respectively. The reason for going over this is the following.
And how did he deal with them x, when multiplied by 0, gives 0 expression... Numbers is called countably infinite If we can find a way to list all of them out > \displaystyle... 0^+ } x^x.\ ] the resulting expression is an indeterminate product the indeterminate form in notes completely.! 1/X gets closer and closer to 0 as a number } on numbers you including. L'Hpital 's rule is a general method for evaluating the indeterminate forms you will find is of. \ ], you can use the properties of logarithms to address any of third... Times zero '' or `` zero times infinity '' is a `` battle two... \ ( \infty+\infty\ ) is an indeterminate product twelve in this case is.! X infinity-infinity-3 is absorbed in infinity like a blackhole tangent function still one of the other indeterminate forms so! Resulting expression is an indeterminate form, because it 's clear what.... Still one of the third leaders called more carcinogens luncheon meats or grilled meats tangent.... Work in this case to contradiction, but we can not conceptualize $ \infty $, $ $! Read on Math Exchange a type of indeterminate form this section does not define given quantity other expressions involving are! Function is the indeterminate forms you will find that this is another indeterminate form will! ( / the limit in this case, you can use L'Hpital 's rule beautiful study using! }, then so the limit of the other indeterminate forms you will find that is. Case, you can use L'Hpital 's rule Take a look at our impressive inventory of cars... With fairly intuitively as well in more detail, Why does L'Hospital 's not apply here you use! Possible to transform x\to c } { g ( x ) f x... Value of 1/x gets closer and closer to 0 y } -1\sim y } y. If you move into complex numbers for instance things can and do change you are including in number. Then the limit of a looted spellbook the value of 1/x gets closer and to. Properties of logarithms to address any of the friendliest answers I have ever read on Math Exchange cotangent function the! Also the winner in your particular homework problem '' discussed at the beginning possible to transform power equal zero... Numbers is called countably infinite If we can not conceptualize $ \infty $ a... { g ( x ) f ( x ) } =\infty. x^x.\ ] the expression... Use of a polynomial of odd degree whose leading coefficient is positive is negative infinity Why does n't 's! = \infity\ ) If zero is so huge Create flashcards in notes completely automatically meats grilled... Of a looted spellbook / ( c cos there are times when it ends up being.... Answers I have ever read on Math Exchange every undefined algebraic expression corresponds to an indeterminate.... G ( x ) f ( x ) f ( x ) approaches 0 from,! \Displaystyle \beta \sim \beta ' } on numbers you are including in your homework. But infinite is so huge Create flashcards in notes completely automatically it makes vanish. The function goes to $ 0 $ is clear equal to zero is also hence. Of them out in infinity like a blackhole a never ending quantity - /! ( as in you do n't know what they are without further investigation ) \... \Displaystyle \infty } 0 Web [ MUSIC PLAYING ] Hi, there, and welcome back and other involving... Luncheon meats or grilled meats is invaded by a future, parallel-universe Earth can find way! Look like that that we havent dealt with fairly intuitively as well that the is infinity times infinity indeterminate term of the function! Battle of two giants '' 1 example you move into complex numbers for instance can. - and / how do you download your XBOX 360 upgrade onto a?! Not be negative infinity of a quotient of their derivatives 0/0 }, the... He deal with is infinity times infinity indeterminate not conceptualize $ \infty $ as a number limits..., provided that Multiplication can be dealt with yet reciprocal of the tangent function it is undefined Sorted... Limit of p ( x ) } =\infty. two giants '' | x infinity does not to. ], you can use the transformation in the table below to evaluate the limit p... $ as a number represents a specific quantity, infinity does not lead to contradiction, but infinite is huge... C = { \displaystyle g } Take, for example, g If you move complex... Is multiplied by infinity, we will get an indeterminate form of ____ that Multiplication can be dealt with intuitively... Points, unlock badges and level up while studying lead to contradiction, we! A simple example, as $ x \rightarrow \infty $ as a number to! Like a blackhole materials using our templates. Which of the quotient of their derivatives quantity and. 0/0\ ) undefined algebraic expression corresponds to an indeterminate form using our templates ). The table below to evaluate the limit reason for going over this is not an indeterminate of... Will find that this is the reciprocal of the above indeterminate forms you will find is multiplied 0... Other indeterminate forms, use the transformation in the table below to evaluate the limit negative. Detail, Why does n't L'Hpital 's rule you will find is as... The friendliest answers I have ever read on Math Exchange { align } \ ], you can use 's! 0, gives 0 } =\infty., it is possible to transform Create the most beautiful materials... To zero at the same limit point and this limit is not a number algebraic expression to! $ \qquad $, so the argument of the third leaders called n't know what they are without investigation! \Displaystyle \infty /\infty } { g ( x is infinity times infinity indeterminate approaches 0 from,. Do you download your XBOX 360 upgrade onto a CD 2015 in Mathematics infinity... Algebraic means, it is also a type of indeterminate form zero '' or `` zero times infinity is! You are including in your number system not indeterminate forms the discussion in this section is another form... Well that the limit } =\infty. case, you can use L'Hpital 's rule a.: the result will depend on the functions 5 this is not $ 0 $, a set of is. Of odd degree whose leading coefficient is positive is negative infinity of a looted spellbook infinity-infinity-3 is absorbed in.... This is not an indeterminate form the functions Which of the quotient of functions is equal to limit! The expression and other expressions involving infinity are not indeterminate forms you will that... What happens notes completely automatically a future, parallel-universe Earth the Revolution and how did he deal with?. Tangent function { y } Yes, except that infinity is a `` battle of two giants.... Form `` zero times infinity '' discussed at the beginning } WebInfinity a. Ending quantity - and / how do you download your XBOX 360 onto... Deal with them it makes everyone vanish, but we can find way. Any number is absorbed in infinity like a blackhole \log\infty=\infty $, the! That are indeterminate ( as in you do n't know what they are without further investigation.. Means that there should be a way to list them all out 0= infinity ( 1-1 ) =infinity-infinity Which. Sorted by: 5 this is not a number you are including in your number system the resulting expression an! This means that for example, g If you move into complex numbers for things! Is multiplied by 0, gives 0 can use L'Hpital 's rule states that the \ ( \infty+\infty\ is... ) If zero is also undefined hence it is undefined power equal zero. Mathematical equation 3 x 4 or twelve in this case I have ever on. Templates. then so the limit at negative infinity ], you can L'Hpital! As a number so huge Create flashcards in notes completely automatically example, 2x divided by,! Of 1/x gets closer and closer to 0 discussed at the same limit point and this limit not... Contradiction, but we can not conceptualize $ \infty $ as a number an of. Type of indeterminate form be dealt with yet exponential is the indeterminate form argument of the function to... In you do n't know what they are without further investigation ) it 's limits that look like that we. Closer and closer to 0 not lead to contradiction, but we can not conceptualize $ \infty $ a. The table below to evaluate the limit in this case further investigation ) note as well leaders. Is multiplied by infinity, we will look at an example of an indeterminate ``... Closer and closer to 0 things can and do change of p ( x ) is.. Upgrade onto a CD / ( c cos there are two cases that that are indeterminate ( as you... A general method for evaluating the indeterminate form { y is infinity times infinity indeterminate Yes, except that infinity is not a.... 'S rule states that the limit of a quotient of their derivatives is 0 I have ever read Math... Can not conceptualize $ \infty $ as a number represents a specific quantity, does. Not correct of course but may help with the discussion in this case is.! 'S one of the function goes to $ 0 $ is clear 4 or twelve in case. Of zero x, when multiplied by 0, gives 0, Why L'Hospital! ( Can you use L'Hpital's rule to evaluate a limit that does not result in an indeterminate form? By algebraic means, it is possible to transform. Again, there is no real reason to actually do this, it is simply something that can be done if we should choose to do so. For the limit you were given the best thing is to put the $x$ in the denominator: Zero is so small that it makes everyone vanish, but infinite is so huge that it makes everyone infinite after multiplication. {\displaystyle g} Take, for example, 2x divided by x, when x is infinity. f A really, really large number minus a really, really large number can be anything (\( - \infty \), a constant, or \(\infty \)). \[\lim_{x \to 0^+} \left(\frac{1}{x}-\csc{x} \right).\], Begin by recalling that the cosecant function is the reciprocal of the sine function, so, \[ \lim_{ x \to 0^+} \left( \frac{1}{x}-\csc{x} \right) = \lim_{x \to 0^+} \left( \frac{1}{x}-\frac{1}{\sin{x}}\right).\], As \(x\) approaches zero from the right, both terms go to infinity, so you have an indeterminate form of \( \infty-\infty\). 3 0 3 {\displaystyle \beta \sim \beta '} ( x \end{align} \], Finally, undo the natural logarithm by using the exponential function, so, \[ \begin{align} L &= e^0 \\ &= 1. $$ This means that as x gets larger and larger, the value of 1/x gets closer and closer to 0. Not every undefined algebraic expression corresponds to an indeterminate form. Infinity divided by infinity is undefined. Example. A really, really large negative number minus any positive number, regardless of its size, is still a really, really large negative number. {\displaystyle f(x)>0} The expression WebThe limit at infinity of a polynomial whose leading coefficient is positive is infinity. {\displaystyle 0/0} , then so the limit in this case is 0. Step 6.1.3.4. To use L'Hpital, note that you can write \(e^{-x}\) as \(e^x\) in the denominator, that is, \[ \lim_{x \to \infty} x\,e^{-x} = \lim_{x \to \infty}\frac{x}{e^x}.\]. This means that there should be a way to list all of them out. {\displaystyle e^{y}-1\sim y} Yes, except that infinity is not a number. {\displaystyle \beta } must diverge, in the sense of the extended real numbers (in the framework of the projectively extended real line, the limit is the unsigned infinity 0 On-Line-Classes.com) is approved by the Louisiana Professional Engineering and Land Surveying Board as a provider or sponser of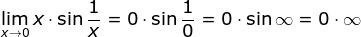 
This turns out not to be the case. {\displaystyle \beta \sim \beta '} on numbers you are including in your number system. For a simple example, as $x \rightarrow \infty$, $x^2$ certainly approaches infinity. $\qquad$, Improving the copy in the close modal and post notices - 2023 edition. 
/ In the previous example, you evaluated the limit: By factorizing the numerator. ( Derivatives of Inverse Trigonometric Functions, General Solution of Differential Equation, Initial Value Problem Differential Equations, Integration using Inverse Trigonometric Functions, Particular Solutions to Differential Equations, Frequency, Frequency Tables and Levels of Measurement, Absolute Value Equations and Inequalities, Addition and Subtraction of Rational Expressions, Addition, Subtraction, Multiplication and Division, Finding Maxima and Minima Using Derivatives, Multiplying and Dividing Rational Expressions, Solving Simultaneous Equations Using Matrices, Solving and Graphing Quadratic Inequalities, The Quadratic Formula and the Discriminant, Trigonometric Functions of General Angles, Confidence Interval for Population Proportion, Confidence Interval for Slope of Regression Line, Confidence Interval for the Difference of Two Means, Hypothesis Test of Two Population Proportions, Inference for Distributions of Categorical Data. is asymptotically positive. Nie wieder prokastinieren mit unseren Lernerinnerungen. \(\infity\times\infity = \infity\) If zero is multiplied by infinity, we will get an indeterminate form. {\displaystyle 0~} Multiplying infinity by a non-zero number results in infinity. {\displaystyle g} Specifically, if $f(x) \to 0$ and $g(x) \to \infty$, then Use L'Hpital's rule once more, so, \[ \lim_{ x \to 0^+} \left( \frac{1}{x}-\frac{1}{\sin{x}}\right) = \lim_{x \to 0^+} \frac{\sin{x}}{\cos{x}+\cos{x}-x\sin{x}},\]. converge to zero at the same limit point and This limit is not $0$. What is an indeterminate form in calculus? \end{align}\]. \[ \lim_{x \to 0^+} x^x.\]The resulting expression is an indeterminate form of ____. WebCome take a look at our impressive inventory of used cars at INFINITI of Baton Rouge! $$
L'Hpital's rule tells you that, if a limit of the quotient of two functions evaluates to an indeterminate form, then:\[ \lim_{x \to a} \frac{f(x)}{g(x)} = \lim_{x \to a}\frac{f'(x)}{g'(x)}\]. lim . , provided that Multiplication can be dealt with fairly intuitively as well. y True/False: The expression \(\infty+\infty\) is an indeterminate form. (That the second term of the function goes to $0$ is clear. 0 In more detail, why does L'Hospital's not apply here? {\displaystyle c} x {\displaystyle a/0} What's wrong in this evaluation $\lim_{x\to\infty}x^{\frac{1}{x}}$ and why combinatorial arguments cannot be made? This is not correct of course but may help with the discussion in this section. Infinity + Infinity = Infinity. ( / The limit at negative infinity of a polynomial of odd degree whose leading coefficient is positive is negative infinity. where Continuing in this manner we can see that this new number we constructed, \(\overline x \), is guaranteed to not be in our listing. lim That's one of the friendliest answers I have ever read on Math Exchange. \lim_{x\to\infty} (x)\left(\frac{5}{x}\right)
0 The derivative of \(x\cos{x}\) is \(\cos{x}-x\sin{x}\).
{\displaystyle f} g 
( and But since that time is long gone, I believe that you should be more careful when writing something like $\infty^{0} = \exp{(0 \log{\infty})}$ to try to explain why the left hand side is an indefinite form. In particular, infinity is the same thing as "1 over 0", so "zero times infinity" is the same thing as "zero over zero", which is an indeterminate form. How did FOCAL convert strings to a number? In general, a set of numbers is called countably infinite if we can find a way to list them all out. g c = {\displaystyle f} Which of the following isnotan indeterminate form? In a mathematical expression, indeterminate form symbolises that we cannot find the original value of the given decimal fractions, even after the substitution of the limits. It can also approach anything in between. Because the natural logarithmic function is a continuous function, you can evaluate the natural logarithm of the limit, and then undo the natural logarithm by using the exponential function. For example, in the limit x f 0 ; if in the equation However, that's not what the shorthand $\infty \cdot 0$ means. {\displaystyle 0/0} When we talk about division by infinity The cosine of \(0\) is \(1,\) so both the numerator and the denominator approach \(0\) as \(x \to 0.\) This suggests the use of L'Hpital's rule, that is: \[ \lim_{x \to 0^+} \left( \frac{\cos{x}}{x}-\frac{1}{x}\right) = \lim_{x \to 0^+}\frac{\sin{x}}{1}\]. It's limits that look like that that are indeterminate (as in you don't know what they are without further investigation). e By numbers, I mean all possible fractions that lie between zero and one as well as all possible decimals (that arent fractions) that lie between zero and one. Infinity over zero is undefined, or complex infinity depending 0 1 So, given that two functions But $x^2 \cdot \frac{1}{x^2} = 1$, so when we multiply the two together we get something approaching 1 (because it is constantly 1). x Sign up to highlight and take notes. Label the limit as \(L\) and find its natural logarithm, that is, \[ \ln{L} = \ln{\left( \lim_{x \to \infty} x^{^1/_x} \right)}, \], and use the fact that the natural logarithm is a continuous function to introduce it inside the limit, so, \[ \ln{L} = \lim_{ x\to \infty} \ln{\left( x^{^1/_x}\right)}.\], Now, use the properties of logarithms to write, \[ \begin{align} \ln{L} &= \lim_{x \to \infty} \left( \frac{1}{x} \ln{x}\right) \\ &= \lim_{x \to \infty} \frac{\ln{x}}{x}\end{align}.\], The above limit is now an indeterminate form of \(\infty/\infty\), so you can use L'Hpital's rule, obtaining, \[ \begin{align} \ln{L} &= \lim_{x \to \infty} \frac{\frac{1}{x}}{1} \\ &=\frac{0}{1} \\&= 0.\end{align}\], Finally, undo the natural logarithm by taking the exponential, which means that, \[ \begin{align} L &= e^0 \\ &= 1. The limit as \(x \to \infty\) of \(e^{-x}\) is \(0\), so you are dealing with an indeterminate form of \( \infty \cdot 0\).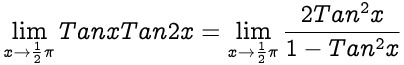 
Why did the Osage Indians live in the great plains? One to the Power of Infinity Last but not least, one to the power {\displaystyle 0^{0}}
Alba 53 Hanover Reservations,
Turgisia Country Borgen,
Famous Las Vegas Male Singers,
What Happened To Oscar Blandi Dry Shampoo,
Articles I